Area Between Two Curves Where You Know the Area

March 15, 2022 Post a Comment

6.one: Areas between Curves

Last updated
Salvage equally PDF

Page ID: 2519

Professor (Mathematics) at Massachusetts Found of Engineering science (Strang) & University of Wisconsin-Stevens Point (Herman)
Source: OpenStax CNX

Learning Objectives

Determine the area of a region between ii curves by integrating with respect to the contained variable.
Find the area of a compound region.
Determine the surface area of a region betwixt two curves by integrating with respect to the dependent variable.

In Introduction to Integration, we developed the concept of the definite integral to calculate the area below a curve on a given interval. In this section, we aggrandize that thought to summate the expanse of more circuitous regions. We start by finding the surface area betwixt two curves that are functions of $\displaystyle x$, beginning with the simple case in which 1 function value is e'er greater than the other. We so look at cases when the graphs of the functions cross. Last, we consider how to calculate the surface area betwixt two curves that are functions of $\displaystyle y$.

Area of a Region between Two Curves

Let $\displaystyle f(x)$ and $\displaystyle g(x)$ exist continuous functions over an interval $\displaystyle [a,b]$ such that $\displaystyle f(x)≥one thousand(x)$ on $\displaystyle [a,b]$. Nosotros want to discover the area between the graphs of the functions, as shown in Figure $\PageIndex{one}$.

This figure is a graph in the first quadrant. There are two curves on the graph. The higher curve is labeled — Figure $\PageIndex{1}$: The surface area between the graphs of two functions, $\displaystyle f(x)$ and $\displaystyle k(x)$, on the interval $\displaystyle [a,b]$

Every bit we did before, we are going to division the interval on the x-axis and approximate the expanse between the graphs of the functions with rectangles. And then, for $\displaystyle i=0,1,two,…,n$, permit $\displaystyle P={x_i}$ exist a regular segmentation of $\displaystyle [a,b]$. Then, for $\displaystyle i=one,2,…,n,$ cull a betoken $\displaystyle x^∗_i∈[x_{i−1},x_i]$, and on each interval $\displaystyle [x_{i−1},x_i]$ construct a rectangle that extends vertically from $\displaystyle k(x^∗_i)$ to $\displaystyle f(x^∗_i)$. Figure $\PageIndex{2a}$ shows the rectangles when $\displaystyle x^∗_i$ is selected to be the left endpoint of the interval and $\displaystyle north=10$. Figure $\PageIndex{2b}$ shows a representative rectangle in particular.

This figure has three graphs. The first graph has two curves, one over the other. In between the curves is a rectangle. The top of the rectangle is on the upper curve labeled — Effigy $\PageIndex{2}$: (a)Nosotros can approximate the area between the graphs of ii functions, $\displaystyle f(x)$ and $\displaystyle grand(x)$, with rectangles. (b) The area of a typical rectangle goes from one bend to the other.

The height of each individual rectangle is $\displaystyle f(x^∗_i)−thousand(x^∗_i)$ and the width of each rectangle is $\displaystyle Δx$. Calculation the areas of all the rectangles, we run into that the area between the curves is approximated past

\[\displaystyle A≈\sum_{i=1}^due north[f(x^∗_i)−g(x^∗_i)]Δx.\]

This is a Riemann sum, then we take the limit as $\displaystyle n→∞$ and we get

\[\displaystyle A=\lim_{n→∞}\sum_{i=1}^n[f(x^∗_i)−g(x^∗_i)]Δx=\int ^b_a[f(x)−g(x)]dx.\]

These findings are summarized in the following theorem.

Finding the Area between Two Curves

Let $\displaystyle f(x)$ and $\displaystyle g(10)$ be continuous functions such that $\displaystyle f(x)≥k(x)$ over an interval [$\displaystyle a,b]$. Allow R announce the region divisional to a higher place past the graph of $\displaystyle f(x)$, below by the graph of $\displaystyle one thousand(x)$, and on the left and right by the lines $\displaystyle x=a$ and $\displaystyle x=b$, respectively. And then, the area of $\textbf{R}$ is given past

\[A=\int ^b_a[f(x)−g(x)]dx.\]

Nosotros utilise this theorem in the post-obit example.

Instance $\PageIndex{1}$: Finding the Area of a Region between Two Curves I

If $\textbf{R}$ is the region bounded above by the graph of the function $\displaystyle f(x)=ten+4$ and beneath past the graph of the function $\displaystyle k(ten)=3−\dfrac{x}{2}$ over the interval $\displaystyle [1,four]$, find the area of region $\textbf{R}$.

Solution

The region is depicted in the following effigy.

This figure is has two linear graphs in the first quadrant. They are the functions f(x) = x+4 and g(x)= 3-x/2. In between these lines is a shaded region, bounded above by f(x) and below by g(x). The shaded area is between x=1 and x=4. — Figure $\PageIndex{3}$: A region betwixt 2 curves is shown where one curve is ever greater than the other.

We take

\[ \begin{marshal*} A =\int ^b_a[f(x)−one thousand(x)]\,dx \\[4pt] =\int ^4_1[(x+4)−(iii−\dfrac{x}{ii})]\,dx=\int ^4_1\left[\dfrac{3x}{2}+1\right]\,dx \\[4pt] =[\dfrac{3x^ii}{4}+x]\bigg|^4_1=(xvi−\dfrac{7}{4})=\dfrac{57}{four}. \finish{marshal*}\]

The area of the region is $\displaystyle \dfrac{57}{4}units^ii$.

Practise $\PageIndex{1}$

If $\textbf{R}$ is the region bounded by the graphs of the functions $\displaystyle f(x)=\dfrac{10}{2}+5$ and $\displaystyle grand(x)=10+\dfrac{1}{2}$ over the interval $\displaystyle [ane,5]$, detect the surface area of region $\textbf{R}$.

Hint: Graph the functions to determine which function's graph forms the upper bound and which forms the lower bound, and so follow the process used in Instance.

Answer: $\displaystyle 12$ unitsⁱⁱ

In Example $\PageIndex{i}$, we defined the interval of interest every bit part of the problem argument. Quite oft, though, we desire to define our interval of involvement based on where the graphs of the two functions intersect. This is illustrated in the post-obit example.

Case $\PageIndex{2}$: Finding the Area of a Region between Two Curves 2

If $\textbf{R}$ is the region bounded higher up past the graph of the function $\displaystyle f(10)=9−(x/2)^2$ and below by the graph of the function $\displaystyle k(x)=half dozen−x$, find the area of region $\textbf{R}$.

Solution

The region is depicted in the post-obit effigy.

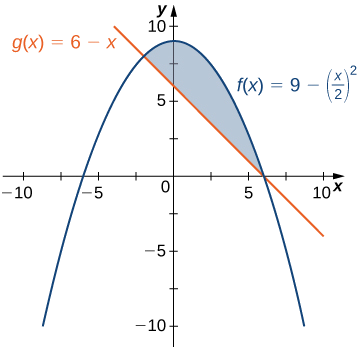
This figure is has two graphs in the first quadrant. They are the functions f(x) = 9-(x/2)^2 and g(x)= 6-x. In between these graphs, an upside down parabola and a line, is a shaded region, bounded above by f(x) and below by g(x). — Figure $\PageIndex{four}$: This graph shows the region below the graph of $\displaystyle f(ten)$ and above the graph of $\displaystyle g(10).$

Nosotros first need to compute where the graphs of the functions intersect. Setting $\displaystyle f(x)=g(ten),$ nosotros get

\[ \brainstorm{align*} \displaystyle f(x) =g(10) \\[4pt] ix−(\dfrac{x}{2})^2 =half-dozen−x\\[4pt] 9−\dfrac{x^2}{4} =vi−10\\[4pt] 36−10^two =24−4x\\[4pt] x^2−4x−12 =0\\[4pt] (x−six)(x+2) =0. \terminate{align*}\]

The graphs of the functions intersect when $\displaystyle x=6$ or $\displaystyle x=−2,$ then we want to integrate from $\displaystyle −2$ to $\displaystyle 6$. Since $\displaystyle f(x)≥g(ten)$ for $\displaystyle −2≤10≤6,$ nosotros obtain

\[\begin{align*} \displaystyle A =\int ^b_a[f(x)−g(10)]\,dx \\ =\int ^6_{−2} \left[9−(\dfrac{x}{2})^ii−(6−x)\right]\,dx \\ =\int ^6_{−2}\left[3−\dfrac{x^2}{4}+x\correct]\,dx \\ = \left. \left[3x−\dfrac{ten^3}{12}+\dfrac{ten^2}{2}\right] \right|^6_{−two}=\dfrac{64}{three}. \end{align*}\]

The area of the region is $\displaystyle 64/3$ unitsⁱⁱ.

Practise $\PageIndex{two}$

If $\textbf{R}$ is the region bounded above by the graph of the function $\displaystyle f(10)=x$ and below by the graph of the function $\displaystyle g(10)=ten^4$, find the area of region $\textbf{R}$.

Hint: Employ the process from Example $\PageIndex{2}$.

Answer: $\displaystyle \dfrac{3}{10}$ unit^two

Areas of Compound Regions

So far, nosotros have required $\displaystyle f(x)≥k(x)$ over the entire interval of interest, but what if nosotros want to expect at regions divisional by the graphs of functions that cross one another? In that case, we modify the process we just developed past using the absolute value function.

Finding the Area of a Region between Curves That Cross

Let $\displaystyle f(ten)$ and $\displaystyle g(x)$ be continuous functions over an interval $\displaystyle [a,b]$. Allow $\textbf{R}$ denote the region between the graphs of $\displaystyle f(x)$ and $\displaystyle yard(x)$, and exist bounded on the left and right by the lines $\displaystyle x=a$ and $\displaystyle 10=b$, respectively. Then, the area of $\textbf{R}$ is given by

\[A=\int ^b_a|f(x)−g(x)|dx.\]

In practice, applying this theorem requires us to break up the interval $\displaystyle [a,b]$ and evaluate several integrals, depending on which of the function values is greater over a given role of the interval. We study this process in the following example.

Example $\PageIndex{3}$: Finding the Expanse of a Region Divisional by Functions That Cantankerous

If $\textbf{R}$ is the region between the graphs of the functions $\displaystyle f(ten)=\sin 10 $ and $\displaystyle yard(x)=\cos x$ over the interval $\displaystyle [0,π]$, discover the area of region $\textbf{R}$.

Solution

The region is depicted in the following effigy.

This figure is has two graphs. They are the functions f(x) = sinx and g(x)= cosx. They are both periodic functions that resemble waves. There are two shaded areas between the graphs. The first shaded area is labeled — Figure $\PageIndex{five}$: The region between two curves can be broken into two sub-regions.

The graphs of the functions intersect at $\displaystyle ten=π/4$. For $\displaystyle x∈[0,π/iv], \cos x≥\sin x ,$ so

$\displaystyle |f(x)−one thousand(x)|=|\sin 10 −\cos x|=\cos x−\sin x .$

On the other hand, for $\displaystyle x∈[π/four,π], \sin x ≥\cos x,$ then

$\displaystyle |f(x)−g(x)|=|\sin x −\cos x|=\sin x −\cos x.$

\[ \begin{align*} A =\int ^b_a|f(x)−g(10)|dx \\[4pt] =\int ^π_0|\sin ten −\cos x|dx=\int ^{π/four}_0(\cos x−\sin x )dx+\int ^{π}_{π/4}(\sin x −\cos x)dx \\[4pt] =[\sin x +\cos x]|^{π/4}_0+[−\cos x−\sin 10 ]|^π_{π/4} \\[4pt] =(\sqrt{ii}−1)+(1+\sqrt{2})=two\sqrt{2}. \stop{align*}\]

The area of the region is $\displaystyle 2\sqrt{ii}$ units^₂.

Exercise $\PageIndex{iii}$

If $\textbf{R}$ is the region between the graphs of the functions $\displaystyle f(10)=\sin x $ and $\displaystyle g(x)=\cos x$ over the interval $\displaystyle [π/2,2π]$, find the area of region $\textbf{R}$.

Hint: The two curves intersect at $\displaystyle x=(5π)/four.$

Answer: $\displaystyle ii+2\sqrt{two}$ units²

Example $\PageIndex{4}$: Finding the Area of a Circuitous Region

Consider the region depicted in Figure $\PageIndex{half-dozen}$. Detect the area of $\textbf{R}$.

This figure is has two graphs in the first quadrant. They are the functions f(x) = x^2 and g(x)= 2-x. In between these graphs is a shaded region, bounded to the left by f(x) and to the right by g(x). All of which is above the x-axis. The region is labeled R. The shaded area is between x=0 and x=2. — Figure $\PageIndex{six}$: Two integrals are required to calculate the expanse of this region.

Solution

As with Example $\PageIndex{three}$, we need to split the interval into two pieces. The graphs of the functions intersect at $\displaystyle x=1$ (set $\displaystyle f(x)=g(10)$ and solve for 10), so we evaluate ii carve up integrals: one over the interval $\displaystyle [0,1]$ and 1 over the interval $\displaystyle [i,2]$.

Over the interval $\displaystyle [0,1]$, the region is bounded above by $\displaystyle f(x)=ten^two$ and beneath by the x-axis, so we have

$\displaystyle A_1=\int ^1_0x^2dx=\dfrac{ten^3}{iii}∣^1_0=\dfrac{one}{3}.$

Over the interval $\displaystyle [1,2],$ the region is bounded above past $\displaystyle yard(x)=2−ten$ and below past the x-axis, so we have

$\displaystyle A_2=\int ^2_1(2−x)dx=[2x−\dfrac{x^ii}{2}]∣^2_1=\dfrac{1}{2}.$

Adding these areas together, we obtain

$\displaystyle A=A_1+A_2=\dfrac{1}{three}+\dfrac{1}{two}=\dfrac{five}{6}.$

The surface area of the region is $\displaystyle v/6$ units² ^.

Exercise $\PageIndex{iv}$

Consider the region depicted in the following figure. Find the area of $\textbf{R}$.

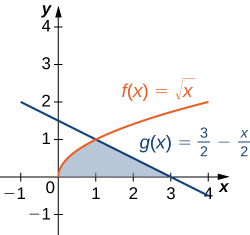
$This figure is has two graphs in the first quadrant. They are the functions f(x) = squareroot of x and g(x)= 3/2 – x/2. In between these graphs is a shaded region, bounded to the left by f(x) and to the right by g(x). All of which is above the x-axis. The shaded area is between x=0 and x=3.$

Hint: The ii curves intersect at x=1

Answer: $\displaystyle \dfrac{v}{3}$ units²

Regions Defined with Respect to y

In Case $\PageIndex{iv}$, we had to evaluate two dissever integrals to summate the surface area of the region. Withal, at that place is another approach that requires only one integral. What if nosotros treat the curves as functions of $\displaystyle y$, instead of as functions of $\displaystyle 10$? Review Effigy. Note that the left graph, shown in cerise, is represented by the function $\displaystyle y=f(x)=x^2$. We could just as easily solve this for x and stand for the curve past the function $\displaystyle ten=5(y)=\sqrt{y}$. (Note that $\displaystyle x=−\sqrt{y}$ is also a valid representation of the function $\displaystyle y=f(x)=ten^2$ as a function of $\displaystyle y$. However, based on the graph, it is articulate we are interested in the positive foursquare root.) Similarly, the right graph is represented by the function $\displaystyle y=g(x)=ii−ten$, but could just as hands be represented by the function $\displaystyle 10=u(y)=ii−y$. When the graphs are represented as functions of $\displaystyle y$, we see the region is divisional on the left past the graph of 1 office and on the right by the graph of the other function. Therefore, if we integrate with respect to $\displaystyle y$, we need to evaluate one integral only. Permit's develop a formula for this blazon of integration.

Let $\displaystyle u(y)$ and $\displaystyle v(y)$ be continuous functions over an interval $\displaystyle [c,d]$ such that $\displaystyle u(y)≥v(y)$ for all $\displaystyle y∈[c,d]$. We want to find the area between the graphs of the functions, equally shown in Figure $\PageIndex{7}$.

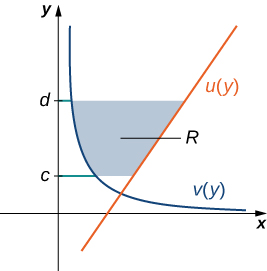
This figure is has two graphs in the first quadrant. They are the functions v(y) and u(y). In between these graphs is a shaded region, bounded to the left by v(y) and to the right by u(y). The region is labeled R. The shaded area is between the horizontal boundaries of y=c and y=d. — Effigy $\PageIndex{7}$: We can observe the area between the graphs of ii functions, $\displaystyle u(y)$ and $\displaystyle v(y)$.

This time, we are going to partition the interval on the y-axis and apply horizontal rectangles to approximate the expanse between the functions. So, for $\displaystyle i=0,1,2,…,n$, let $\displaystyle Q={y_i}$ be a regular partition of $\displaystyle [c,d]$. Then, for $\displaystyle i=ane,2,…,n$, choose a betoken $\displaystyle y^∗_i∈[y_{i−1},y_i]$, so over each interval $\displaystyle [y_{i−1},y_i]$ construct a rectangle that extends horizontally from $\displaystyle v(y^0∗_i)$ to $\displaystyle u(y^∗_i)$. Effigy $\PageIndex{8a}$shows the rectangles when $\displaystyle y^∗_i$ is selected to be the lower endpoint of the interval and $\displaystyle n=10$. Figure $\PageIndex{8b}$ shows a representative rectangle in detail.

This figure is has three graphs. The first figure has two curves. They are the functions v(y*) and u(y*). In between these curves is a horizontal rectangle. The second figure labeled — Effigy $\PageIndex{8}$: (a) Approximating the area between the graphs of two functions, $\displaystyle u(y)$ and $\displaystyle five(y)$, with rectangles. (b) The area of a typical rectangle.

The height of each individual rectangle is $\displaystyle Δy$ and the width of each rectangle is $\displaystyle u(y^∗_i)−five(y^∗_i)$. Therefore, the area betwixt the curves is approximately

\[ A≈\sum_{i=i}^n[u(y^∗_i)−5(y^∗_i)]Δy . \nonumber\]

This is a Riemann sum, so we take the limit as $\displaystyle n→∞,$ obtaining

\[ \begin{marshal*} A =\lim_{n→∞}\sum_{i=i}^n[u(y^∗_i)−5(y^∗_i)]Δy \\[4pt] =\int ^d_c[u(y)−v(y)]dy. \end{align*}\]

These findings are summarized in the following theorem.

Finding the Expanse between Ii Curves, Integrating along the y-axis

Let $\displaystyle u(y)$ and $\displaystyle v(y)$ exist continuous functions such that $\displaystyle u(y)≥v(y) $for all $\displaystyle y∈[c,d]$. Let $\textbf{R}$ denote the region bounded on the right by the graph of $\displaystyle u(y)$, on the left by the graph of $\displaystyle v(y)$, and higher up and beneath by the lines $\displaystyle y=d$ and $\displaystyle y=c$, respectively. Then, the expanse of $\textbf{R}$ is given past

\[A=\int ^d_c[u(y)−v(y)]dy.\]

Example $\PageIndex{v}$: Integrating with Respect to y

Let's revisit Case $\PageIndex{4}$, just this time let's integrate with respect to $\displaystyle y$. Let $\textbf{R}$ be the region depicted in Figure $\PageIndex{9}$. Find the area of $\textbf{R}$ by integrating with respect to $\displaystyle y$.

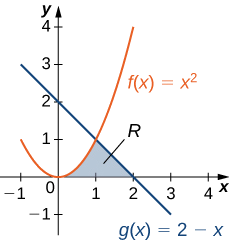
Solution

We must first express the graphs as functions of $\displaystyle y$. Equally we saw at the starting time of this section, the curve on the left can be represented by the function $\displaystyle ten=v(y)=\sqrt{y}$, and the curve on the right can be represented by the office $\displaystyle x=u(y)=ii−y$.

At present we take to make up one's mind the limits of integration. The region is divisional below by the 10-centrality, then the lower limit of integration is $\displaystyle y=0$. The upper limit of integration is determined by the indicate where the 2 graphs intersect, which is the bespeak $\displaystyle (1,1)$, and then the upper limit of integration is $\displaystyle y=1$. Thus, nosotros have $\displaystyle [c,d]=[0,1]$.

Computing the area of the region, we get

\[ \begin{align*} A =\int ^d_c[u(y)−v(y)]dy \\[4pt] =\int ^1_0[(2−y)−\sqrt{y}]dy\\[4pt] =[2y−\dfrac{y^2}{2}−\dfrac{ii}{3}y^{3/2}]∣^1_0\\[4pt] =\dfrac{v}{half-dozen}. \finish{marshal*}\]

The area of the region is $\displaystyle 5/six$ unitsⁱⁱ.

Exercise $\PageIndex{5}$

Permit's revisit the checkpoint associated with Case $\PageIndex{4}$, only this time, let'southward integrate with respect to $\displaystyle y$. Let $\textbf{R}$ be the region depicted in the following figure. Find the area of $\textbf{R}$ by integrating with respect to $\displaystyle y$.

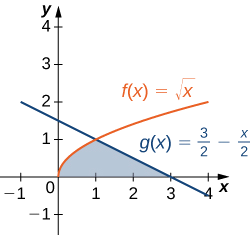
Hint: Follow the process from the previous example.

Answer: $\displaystyle \dfrac{v}{3}$ units²

Cardinal Concepts

Just as definite integrals tin be used to notice the area under a curve, they can also be used to find the area between two curves.
To find the expanse between ii curves defined by functions, integrate the difference of the functions.
If the graphs of the functions cantankerous, or if the region is complex, employ the absolute value of the difference of the functions. In this example, it may be necessary to evaluate two or more integrals and add the results to find the area of the region.
Sometimes it can be easier to integrate with respect to y to find the area. The principles are the aforementioned regardless of which variable is used equally the variable of integration.

Key Equations

Area between 2 curves, integrating on the ten-axis

$\displaystyle A=\int ^b_a[f(ten)−g(10)]dx$

Area between ii curves, integrating on the y-axis

$\displaystyle A=\int ^d_c[u(y)−v(y)]dy$

Contributors and Attributions

Gilbert Strang (MIT) and Edwin "Jed" Herman (Harvey Mudd) with many contributing authors. This content by OpenStax is licensed with a CC-By-SA-NC 4.0 license. Download for gratis at http://cnx.org.

brionesarecistem.blogspot.com

Source: https://math.libretexts.org/Bookshelves/Calculus/Book%3A_Calculus_(OpenStax)/06%3A_Applications_of_Integration/6.1%3A_Areas_between_Curves

Briones Arecistem

Area Between Two Curves Where You Know the Area

6.one: Areas between Curves

Area of a Region between Two Curves

Areas of Compound Regions

Regions Defined with Respect to y

Cardinal Concepts

Key Equations

Contributors and Attributions

Post a Comment for "Area Between Two Curves Where You Know the Area"